Amalia, Defiant Strike, and How to Think About Shuffled Decks

08/18/2024

This mini article is a response to a recent tweet by Matt Nass, which posed an interesting math problem:

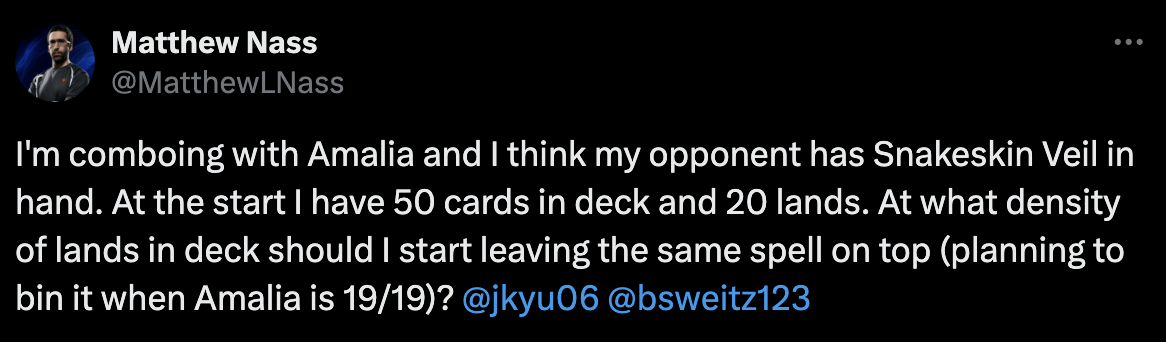
the tweet

I started writing up an answer in Google Docs, and then put enough effort in that I figured I'd just put in on my website for better formatting! So this is that.

Part 1: A Classic “Probability” Question

There’s a classic question in mathematics that is as follows:

Let's play a game.

I have a deck of cards. 26 red cards and 26 black cards. I shuffle the deck, and then say the following: I will reveal cards from the top of the deck one by one, and at ANY point before the last card is revealed (including before I reveal any cards), you can tell me “STOP!” After you tell me to stop, I will reveal the next card - if that card is black, you win; if it’s red, I win. What is your best strategy?

You might think that there’s some complex strategy involving paying attention to what cards are flipped, and calling “STOP” when there are more red cards flipped than black cards. You might be inspired by counting cards in Blackjack, and think that surely there must be some strategy here. But, perhaps surprisingly, the answer is that no strategy can do better than 50%!

You could delve deep into probabilities and algebra, but there’s actually a very elegant proof; one of those proofs that seem so simple once they’re laid out, but require a different framing in such a way that makes them harder to see at first glance. The proof is as follows:

Imagine I propose the same game, but with one change of rules: in this new game, instead of revealing the top card after “STOP”, I reveal the bottom card of the deck. “Now hold on!” you protest. “That’s not a game at all! The bottom card is completely set after you shuffle; it’ll always be a 50/50 chance no matter when I say STOP!”

And that’s true! In this new game, it’s very easy to see why you never have more than a 50% chance at victory. But now, imagine actually playing through that game. Imagine the point where you say “STOP!” Does it actually make a difference whether you reveal the top card or the bottom card at that point? The whole deck is shuffled, so the remainder after stopping is also fully randomized. The top card is no more or less likely to be black than the bottom card - that is to say, they’re both exactly 50/50.

It’s very easy to try to read some strategy into the cards already revealed - surely I can choose to stop when the distribution is better for me, right? But the problem is you always have to stop at some point, and waiting could get you a better distribution, or it could force you into a worse one - and if you calculated all the probabilities and distributions you would find that you can simply never actually affect anything.

Blackjack card-counting works because you can control when and how to bet. A simple game like this one has no room for such agency.

Part 2: Defiant Strike Your Amalia?

Imagine now that you’re playing Pioneer, against an Amalia deck. They’re comboing off with Amalia -

Amalia Benavides Aguirre and

Wildgrowth Walker are both in play and repeatedly triggering each other - and the only relevant instant speed interaction either player has at this point is your single copy of

Defiant Strike. You can cast it at any point, but after it resolves you will have no more mana to cast other spells.

Now your opponent is clever, and knows you have your Defiant Strike in hand. So for a while now, they’ve decided to keep putting the same nonland card on top with Amalia’s explore triggers. But once Amalia goes from 18 to 19 power, they decide to put the nonland into the graveyard. The ball is now in your court.

Amalia will now keep exploring at least until it hits another nonland card. If you never cast your Defiant Strike, that nonland will then cause Amalia to wrath the board, halt the combo, and kill you. So you can’t let that happen - you need to cast your Defiant Strike before Amalia explores a nonland card.

However, when you do cast your Defiant Strike, Amalia’s power will tick up to exactly 20 - so the next card being a nonland will cause the combo to spiral out of control and draw the game (the outcome you want), but the next card being a land will instead cause Amalia to wrath the board, halt the combo, and kill you.

Assuming neither player knows anything about the rest of the deck’s composition (say it was recently shuffled for a

Chord of Calling), when should you cast Defiant Strike, to maximize your chances of drawing the game?

Well, we can compare this to the above situation! Similarly to above, the Amalia player is flipping cards one at a time, until at some point you say “DEFIANT STRIKE!”. Then, once you cast your Defiant Strike, they flip one more card - and if it’s a land you lose, while if it’s a nonland you win. If the game were just that simple, we would know that it doesn’t matter when you cast Defiant Strike - the chance that the card after Defiant Strike is a land are always equivalent to the chance that the bottom card of the deck is a land.

However, there’s one complication here: every time you let the Amalia player explore and reveal a card, you lose on the spot if it’s a nonland. So, each card flipped can only make the game worse for you compared to the previous game, never better. Therefore, the optimal play is now uniquely to use Defiant Strike as soon as possible. And your chance of winning is, again, simply the fraction of nonlands in the deck.

Part 3: The Meta-Game

So, finally, let’s consider the perspective of the Amalia player - the actual question Matt Nass posed in his tweet. You’re playing Amalia, and you want to know when to start keeping the same nonland on top, so you can setup the best possible version of the situation above.

Surely, now that we’re talking up one step in the meta-ness, now that we’re playing a game of games rather than just the base kind of game, things have changed, right? ...right?

Well, not really. Let’s reframe things a bit.

Let's play a game.

You have a deck of lands and nonlands. You will flip cards over, one by one. Any time you flip over a nonland card, you can say “KEEP IT ON TOP NOW!” Once you do, you set up the situation from part 2: your opponent now has to choose when to cast Defiant Strike. And as we know from part 2, the chance of you winning at that point (as opposed to a draw occurring) is simply equal to the fraction of lands remaining in your deck.

So, when should you say “KEEP IT ON TOP NOW!” in order to maximize the fraction of lands remaining in your deck?

…is this starting to sound familiar yet?

As it turns out, the fraction of lands remaining in your deck is… equivalent to the probability that the bottom card of your deck is a land! So, again, no matter what your strategy is, you still can never do better than random.

So, stop whenever! Honestly, stop right away! Technically you gain a small amount of information equity by not showing your opponent more cards than you need to from exploring.

Conclusion

This is a powerful truth about these games involving choosing when to stop flipping cards. It’s so easy to assign meaning to the top card of a deck, to feel like you have agency when you’re given the choice of when to stop flipping cards.

But, you should always remember the unchanging uncertainty of the bottom card.

#FreePalestine | Consider donating to UNWRA or PCRF, supporting protesters locally, and educating yourself.